Introduction to differentiation: Definition of differentiation
The notion of difference quotient
Below you see the graph of the function #f(x)=\frac{1}{10}x^2+5# and the tangent line #l# to #f# at the point #\rv{2,{{27}\over{5}}}#. You can also see the line #m# through #\rv{2, {{27}\over{5}}}# and #\rv{3,{{59}\over{10}}}#. Both points lie on the graph of #f#.
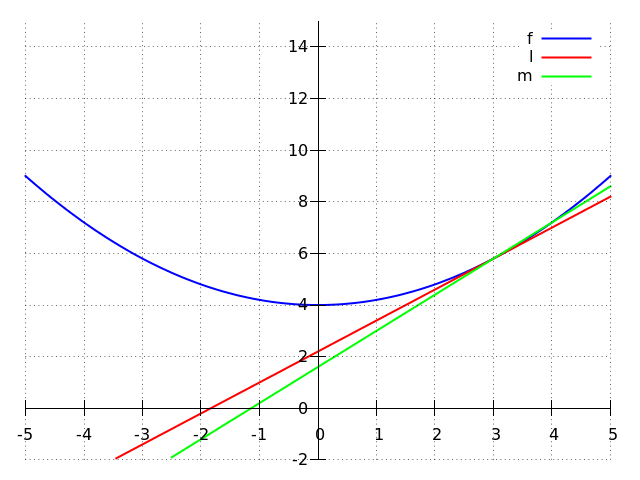
Approximate the slope of the tangent line #l# by calculating the slope of line #m#.
Approximate the slope of the tangent line #l# by calculating the slope of line #m#.
| The slope of the line #m# through #\rv{2, {{27}\over{5}}}# and #\rv{3, {{59}\over{10}}}# is |
Unlock full access
Teacher access
Request a demo account. We will help you get started with our digital learning environment.
Student access
Is your university not a partner?
Get access to our courses via Pass Your Math independent of your university. See pricing and more.
Or visit omptest.org if jou are taking an OMPT exam.
Or visit omptest.org if jou are taking an OMPT exam.
