Linear formulas and equations: Linear functions
 Linear formula
Linear formula
Draw a graph for the formula:
\[y=3\cdot x-6\]
\[y=3\cdot x-6\]
First we create a table for this graph. For this, we substitute the #x#-values in the function. For #x=-4# we find #y=3\cdot \left(-4\right)-6=-18#. Other #x#-values work in the same manner, and can be found in the table below.
To compose the graph, we draw a coordinate system. Next, we draw the points from this table in the coordinate system since these are points of the graph. The #x#-values are shown on the horizontal axis, and the #y#-value on the vertical axis. Next, these points are connected through a straight line. We then get the graph below. The points from the table are drawn in red. Please note that since we know that a linear formula has a straight line as a graph, it is sufficient to draw just two points.
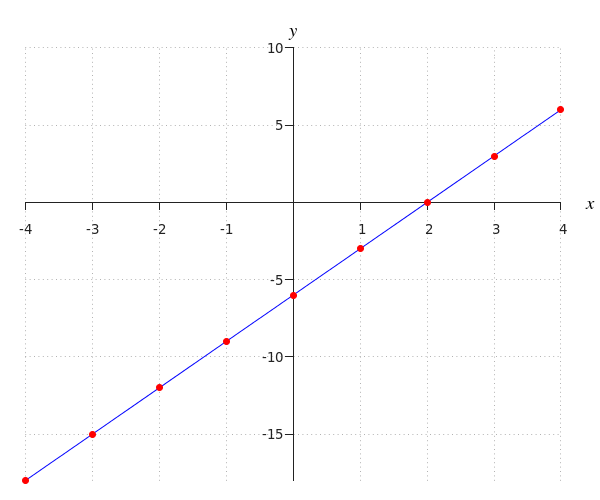
| #x# | #-4# | #-3# | #-2# | #-1# | #0# | #1# | #2# | #3# | #4# |
| #y# | #-18# | #-15# | #-12# | #-9# | #-6# | #-3# | #0# | #3# | #6# |

Unlock full access


Teacher access
Request a demo account. We will help you get started with our digital learning environment.
Student access
Is your university not a partner?
Get access to our courses via Pass Your Math independent of your university. See pricing and more.
Or visit omptest.org if jou are taking an OMPT exam.
Or visit omptest.org if jou are taking an OMPT exam.



