Functions: Power functions
Power functions
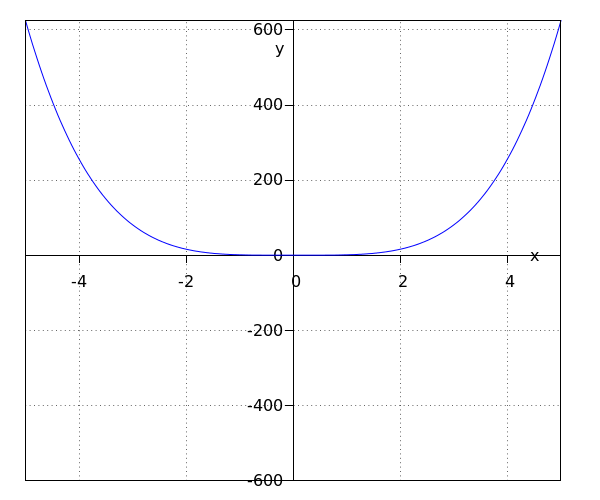
The function #\blue {f}# has the interval #\ivco{0}{\infty}# as range.
The #y#-axis is the symmetry axis.
The vertex is #\rv{0,0}#.
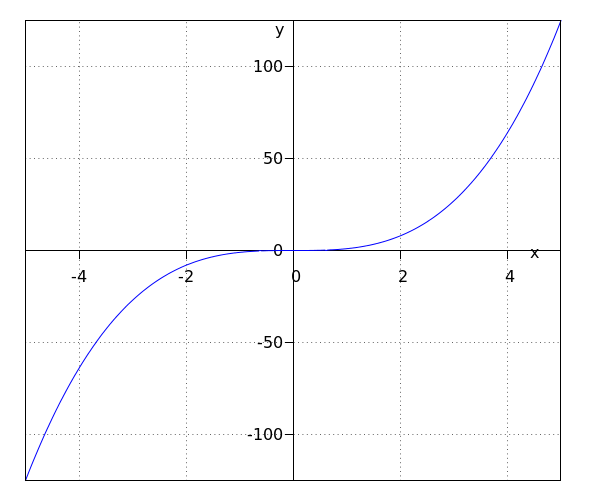
The function #\green{g}# has the interval #\ivoo{-\infty}{\infty}# as range.
The point #\rv{0,0}# is a point of symmetry.
The two functions in the example above are both power functions. It is obvious both functions differ quite a lot. This has got to do with the fact that #\blue{f}# is a power function with an even exponent and that #\green g# is a power function with an odd exponent.
A function of the form \[f(x)=\blue{a}x^{\orange{n}}\] with #\blue{a} \ne 0# is a power function.
The graph of a power function with integer #\orange{n} \gt 0# moves through the points #\rv{0,0}# and #\rv{1,\blue a}#.
Furthermore, the graphs of power functions differ depending on if #\orange{n}# is even or odd. With even #\orange{n}# the graph is symmetrical across the #y#-axis. With odd #\orange{n}# the graph is symmetrical across the point #\rv{0,0}#.
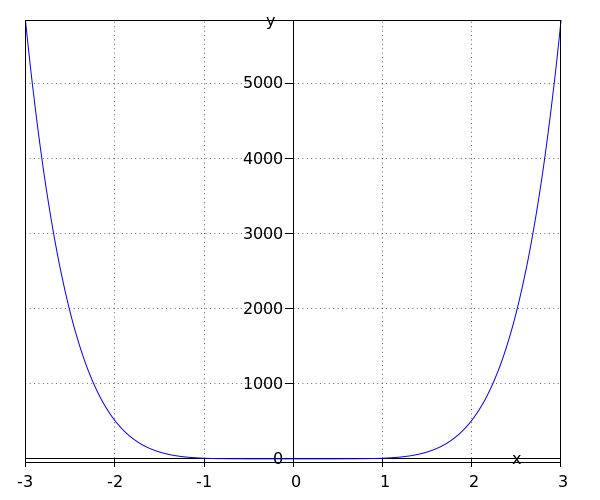
What do we know about the value of #n# and #a#?
The value of #a# is: negative
The graph is symmetrical in the #y#-axis, hence, the value of #n# is even.
The #y#-value is negative if the value of #x# is positive, hence, the value of #a# is negative.
Or visit omptest.org if jou are taking an OMPT exam.
